When is R[x] a principal ideal ring?
Henry Chimal-Dzula∗, C. A.
López-Andradeb
a Ohio University,
Department of Mathematics, Athens, USA.
b Benemérita Universidad Autónoma de Puebla, Facultad de Ciencias
Físico Matemáticas, Puebla, México.
Abstract
Because of its interesting
applications in coding theory, cryptography, and algebraic combinatorics, in
recent decades a lot of attention has been paid to the algebraic structure of
the ring of polynomials R[x], where R is a
finite commutative ring with identity. Motivated by this popularity, in this
paper we determine when R[x] is a
principal ideal ring. In fact, we prove that R[x] is a
principal ideal ring if and only if R is a finite direct product of finite
fields.
Keywords: Principal ideal ring, polynomial ring, finite rings. MSC2010: 13F10, 13F20, 16P10, 13C05.
¿Cuándo R[x] es un anillo de ideales principales?
Resumen
Debido a sus interesantes aplicaciones en teoría de códigos, criptografía y combinatoria algebraica, en décadas recientes se ha incrementado la atención en la estructura algebraica del anillo de polinomios R[x], donde R es un anillo conmutativo finito con identidad. Motivados por esta popularidad, en este artículo determinamos cuándo R[x] es un anillo de ideales principales. De hecho, demostramos que R[x] es un anillo de ideales principales, si y sólo si, R es un producto directo finito de campos finitos.
Palabras clave: Anillo de ideales principales, anillo de polinomios, anillos finitos.
1. Introduction and preliminaries
Polynomials with
coefficients in a finite commutative ring R with identity arise naturally, for
instance, in practical applications dealing with coding theory, cryptography
and algebraic combinatorics, e. g. [1], [2], [3], [4], [6]. For some of these
applications, it is important to know the algebraic structure of either the
ring of polynomials R[x] or the
quotient ring R[x]/A, where A is an ideal of R[x]. In particular, often one wants to know when
such rings are principal ideal rings (PIR’s). Motivated by this question, in
this paper we examine when R[x] is a
PIR. We prove that R[x] is a
principal ideal ring if and only if R is a finite direct product of finite
fields. To this end, let us start remembering some facts about commutative
finite rings with identity.
The most familiar example
of a finite commutative ring with identity is the ring Zm of integers modulo m ≥ 2. When m is a
composite number, the Chinese Remainder Theorem assures that
,
Where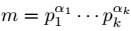 
is the prime factorization of
m, and 
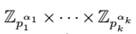is the direct product of the rings 
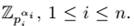Remember that for each 
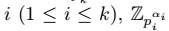is a finite local ring with
maximal ideal hpii (see [5]). In particular, note that
if α = 1, then Zp is a finite field (see [4]).
Consequently, the Chinese Remainder Theorem establishes that Zm is isomorphic to a direct product of
finite local rings. This is the simplest case of the following result.
Theorem 1.1
(Structure of Finite Commutative Rings, [5], Theorem VI.2). Every finite
commutative ring with identity is isomorphic to a direct product of finite
commutative local rings with identity. This decomposition is unique up to the
order of the factors.
In view of the previous
theorem, if R is a finite commutative ring with
identity and R1 ×···× Rn is the unique decomposition of R as a
direct product of local rings, then there exists a ring isomorphism ϕ : R → R1 ×···× Rn which maps each element r ∈ R into a unique n-tuple ϕ(r) = (r1,...,rn) ∈ R1 ×···× Rn.
This map extends to a ring isomorphism
Φ : R[x] → R1[x] ×···× Rn[x],
defined by
Proposition 1.2. Let R1,...,Rn be commutative rings with identity. Then their direct product R = R1 ×···× Rn is a PIR if and only if Ri is
a PIR for each i, 1 ≤ i ≤ n.
Proof. Let A be an
ideal of R and for each i, 1 ≤ i ≤ n, define Ai = {ai ∈ Ri :
(a1,...,ai,...,an) ∈ A}. Then Ai is
an ideal of Ri and we claim that A = A1 ×···×An. The inclusion A ⊆ A1 ×···× An is clear. To prove the reverse
inclusion, let a = (a1,...,an)
be an element in A1 ×···× An. It follows from the definition of Ai that
there exist α1,...,αn ∈ A such that
a = α1e1 +···+αnen,
where ei denotes the element of R with a 1
in the ith coordinate and 0’s elsewhere.
Hence, using that A is an ideal of R1×···×Rn, we have that a ∈ A. Therefore A = A1×···×An, and so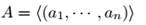 
if and only if Ai = haii for every i, 1 ≤ i ≤ n.
In light of Proposition
1.2 and the isomorphism Φ given in (1), R[x] is a PIR
if and only if Ri[x] is a PIR
for each i, 1 ≤ i ≤ n. Hence, in order to determine
whenever R[x] is
When is R[x] a principal
ideal ring?
a PIR, it is natural to
ask when Ri[x] is a
PIR. This is the purpose of the next section. To illustrate the results of
Section 2, we present in Section 3 three families of finite rings with identity
that are relevant in the theory of finite commutative rings.
2. Ideals of R[x]
First of all, by a ring R we will
always mean a commutative ring with identity 1 6= 0, and remember that a ring R is called
local if it has a unique maximal ideal, or equivalently, R is a local
ring with maximal ideal M if and only if M = R\U(R), where U(R) denotes
the group of units of R. We usually write (R,M) or (R,M,F) to
denote a local ring R, its maximal ideal M and its
residue field F = R/M (using
that M is a maximal ideal of R, the
quotient ring R/M is indeed a field).
The simplest case of a
finite local ring R is when R is a finite field. In this case the
ring R[x] of
polynomials with coefficients in R is a PIR. In fact, R[x] is a principal ideal domain (PID). Therefore,
in what follows we focus our attention on a finite local ring R which is
not a field.
Let (R,M,F) be a
finite local ring which is not a field. Note that the natural surjective
homomorphism − : R → F induces a
surjective polynomial ring homomorphism µ : R[x] → F[x]
given by
.
This ring homomorphism lets us deduce some facts about polynomials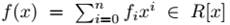 
by using the structure of F[x]. In
particular, note that µ(f(x)) = 0 if and only if fi ∈ M for all i, 0 ≤ i ≤ n and so, it follows that kerµ is a
proper ideal of R[x] (indeed,
kerµ is a prime ideal of R[x] because R[x]/kerµ ∼= F[x]
is an integral domain).
On the other hand,
remember that an element r of a ring R is called
irreducible if it is neither 0 nor a unit in R and the condition r = ab, for some a,b ∈ R, implies
that a ∈ U(R) or b ∈ U(R).
Having remembered the
above, if f(x) ∈ R[x] is such that µ(f(x)) is irreducible in F[x], then f(x) is also
irreducible in R[x]. Since
for every finite field K and every positive integer n there
exists an irreducible polynomial in K[x] of
degree n (see [4]) , we conclude that
irreducible polynomials in R[x] of
degree n exist for every positive integer n. It is
worth to mention that the converse of this fact does not hold in general. That
is, if f(x) is an
irreducible polynomial in R[x] then µ(f(x)) is not
necessarily irreducible in F[x]. For
instance, if p ≥ 2 is a prime number, then p ∈ Zp2[x] is irreducible in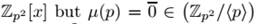
is not irreducible by definition.
Furthermore, if f(x) = x2 + 2x + 3 ∈ Z4[x] then f(x) is irreducible in Z4[x]; whereas
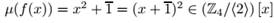is not irreducible.
Lemma 2.1.
Let (R,M) be a local ring which is not a field, p(x) an irreducible polynomial in R[x] and θ ∈ M \{0}.
Then hp(x),θi is a proper ideal of R[x].
Proof. If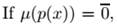
then p(x) ∈ kerµ, so that hp(x),θi ⊆ kerµ $ R[x]. If µ(p(x)) = 6 0, then we
proceed by contradiction. Assume then the existence of some polynomials
H.
Chimal-Dzul & C.A. López-Andrade
f(x),g(x) ∈ R[x]
such that 1 = f(x)θ + g(x)p(x). This
implies that 
which contradicts the irreducibility of p(x). Hence, for every irreducible polynomial p(x) in R[x] and θ ∈ M \{0}, hp(x),θi is a proper ideal of R[x]. X
If p(x) = p0 + p1x + ··· + pnxn ∈ R[x] is an irreducible polynomial in R[x] such that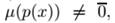 
then deg(µ(p(x))) = k ≥ 1, since µ(p(x)) can not be a unit in F[x]. This implies that pk ∈ U(R) and pk+1,...,pn ∈ M and so, for each nonzero polynomial a(x) ∈ R[x] we have that deg(p(x)a(x)) ≥ k ≥ 1. Consequently, every nonzero polynomial in hp(x)i ⊂ R[x]
is not a constant
polynomial, and so M *hp(x)i.
Theorem 2.2.
Let (R,M) be a local ring which is not a field. Then R[x] is not a PIR.
Proof. Let p(x) ∈ R[x] be an irreducible polynomial in R[x] such that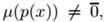 
and let θ ∈ M \{0}.
Then we claim that hp(x),θi is not a principal ideal of R[x]. Assume the contrary; that is, suppose that
there is a polynomial h(x) ∈ R[x] such that hp(x),θi = hh(x)i. Then there exists f(x) ∈ R[x] such that p(x) = f(x)h(x). Using that p(x) is
irreducible, we have that f(x) ∈ U(R[x])
or h(x) ∈ U(R[x]). It
follows immediately from Lemma 2.1 that h(x) ∈/ U(R[x]), and so f(x) ∈ U(R[x]). This implies that hp(x),θi = hh(x)i = hp(x) · f(x)−1i
= hp(x)i, which
is a contradiction since θ ∈h/ p(x)i. In consequence, hp(x),θi is not a principal ideal in R[x]. X
Note that we have proved
implicitly in Theorem 2.2 that for every local ring (R,M,F) which
is not a field, the family of ideals hp(x),θi, where p(x) is an
irreducible element in R[x] such
that 
and θ is a nonzero element in M, consists
entirely of non-principal ideals of R[x]. This family
contains an infinite number of elements since irreducible polynomials of degree
n in R[x] exist
for every integer n ≥ 1.
Theorem 2.3.
Let R be a finite ring. The following
statements are equivalent:
1.
R[x] is a
PIR.
2.
R is isomorphic to a direct product of
finite fields.
Proof. It
follows immediately from Proposition 1.2 and Theorems 1.1, 2.2. X
An equivalent way to state
Theorem 2.3 is as follows: R[x] is a not
a PIR if and only if R is isomorphic to a direct product R1 ×···×Ri×···×Rn of finite local rings such that at least one of
them is not a finite field, say (Ri,Mi,Fi). In this case, for all θ ∈ Mi\{0} and for all irreducible polynomials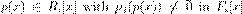 
we have that hp(x),θii⊂ Ri[x] is not a principal ideal in Ri[x]. In consequence, by using the ring isomorphism
Φ defined in
(1), one can easily show that hΦ−1(pi(x)·ei),Φ−1(θi·ei)i⊂
R[x]
is not a principal
ideal in R[x].
Another equivalent way to
state Theorem 2.3 is derived from the structure theorem for commutative PIR’s
due to Zariski-Samuel (see [7, Theorem 33]). This result states that every
commutative PIR is (isomorphic to) a direct sum of PID’s and of special PIR’s.
Therefore, Theorem 2.3 shows that Zariski-Samuel theorem can be expressed for
the ring of polynomials over a finite ring R as follows: R[x] is a PIR if
and only if R[x] is isomorphic to a direct product of PID’s. Moreover, the isomorphism Φ given in (1)
presents one decomposition of R[x] as a
direct product of PID’s. In addition, Theorem 2.2 also implies that for a local
ring (R,M) which is not a field, the ring of polynomials R[x] cannot be a special PIR.
3. Examples
As a first example we
present the one that we used to motivate Theorem 1.1. Let m ≥ 2 be an
integer. Then
where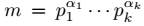 
is the prime factorization of m. Since
is a finite field if and only if αi = 1, it follows from Theorem 2.3 that Zm[x] is a PIR
if and only if α1 = ··· = αk = 1.
In order to generalize the
previous example, let us to consider the ring Zpα, where p is a prime number and α is a
positive integer. Let f(u) be a
monic polynomial of degree r ≥ 1 in Zpα[u] such
that µ(f(u)) is irreducible in (Zpα/hpi)[u]. Then the Galois ring of
characteristic pα and
cardinality pαr is definied as the quotient ring
This ring is a finite
local ring with maximal ideal hp+hf(u)ii, and residue field isomorphic to Zp[u]/hµ(f(u))i (see
[5] for more details). Note that if r = 1 then
.
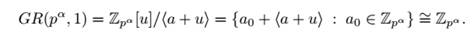Consequently, GR(p,1) ∼= Zp is a finite field, and for any
integer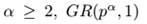 
is a finite local ring which is not
a field. Furtheremore, if r ≥ 2 and α = 1, then GR(p,r) = Zp[u]/hf(u)i is a
finite field with pr elements (see [4]). Hereof it
follows from Theorem 2.2 that
Let p1,p2,...,pk be prime numbers, αi,ri positive integers for 1 ≤ i ≤ k, and
consider the following ring which is a natural generalization of Zm:
.
In analogy with Zm, we deduce from (2) and Theorem 2.3
that R[x] is a PIR
if and only if α1 = ··· = αk = 1. On the other hand, R[x] is not a
PIR if and only if αi ≥ 2 for some i, 1 ≤ i ≤ k.
In the examples given
above we have presented infinite families of finite rings for which the ring of
polynomials with coefficients in these rings are not PIR. The common ground in
both families of rings is that they are finite products of local rings whose
ideals are principal and they are linearly ordered by inclusion. Any local ring
satisfying these conditions is called a finite chain
ring (see [1]). In
general, if R is a finite chain ring that is not a
field, then R[x] is not a
PIR by Theorem 2.2, and so for every finite ring R such that it is isomorphic to a
direct product of finite chain rings (of which at least one is not a field),
the ring R[x] is not a
PIR by Theorem 2.3.
In the following lines, we
are going to present a family of finite local rings which was introduced in
[2].
Let p be a prime
number, α a positive
integer and denote by Fq the unique
finite field with q = pα elements (see [5]). Then for every
integer k ≥ 1, the quotient ring Rk = Fq[u1,u2,...,uk]/hu21,u22,...,u2ki is a commutative ring with identity. Furthermore, it is proved in [2]
that Rk is a finite local ring with maximal
ideal M = h[u1],[u2],...,[uk]i and residue field Rk/M ∼= F2. In
consequence, Theorem 2.3 shows that Rk[x] is not a
PIR for any integer k ≥ 1.
Since k ≥ 2, the ring Rk described
above is neither a PIR nor a chain ring in view of M is not a principal ideal and h[ui]i and h[uj]i are not linearly ordered by
inclusion for i 6= j. However,
it was pointed out in [2] that Rk is a finite
Frobenius ring.
Acknowledgement
We would like to thank the
anonymous referee for his/her useful comments and suggestions that
significantly contributed to improve the quality of our manuscript.
References